A popular misconception is that the Wright brothers, in addition to all of their other achievements, invented the airfoil. They didn’t. Sir John Cayley, an English engineer who also first identified the four forces of flight—lift, drag, thrust and weight—developed the cambered airfoil through detailed experimentation. His three-part work, On Aerial Navigation, published in 1809 and 1810, is often cited as the first description of what we today call an airplane. Also today, we teach that the theories of Sir Isaac Newton (1642-1726) and Swiss mathematician Daniel Bernoulli (1700-1782) provide the detailed science that explains lift. They don’t, at least not fully.
The basic problem is that neither theory completely explains real-world observations. Bernoulli’s principle—that the faster air on top of the wing experiences reduced pressure—is correct but doesn’t explain why it’s correct. It also doesn’t explain inverted flight. That’s where Newton’s second and third laws (see the sidebar below for details) come into play. Taken together, Newton’s laws describe how we can fly inverted and how angle of attack works. But they don’t have the details we need from Bernoulli. Still, once we put Bernoulli and Newton in the same room, then sprinkle some Cayley throughout, we have a working idea of how to build and fly an airplane. But we still don’t know exactly why the air on top of the wing is at a lower pressure than the air underneath it.
Low Pressure Zone
We probably were told in ground school that the low-pressure area on top of the wing results from the air particles passing over it having to accelerate in relation to the air below the wing so that they both arrive at the trailing edge at the same time and rejoin. This is commonly known as the “longer path” or “equal transit time” theory.
But there’s no science that says the air particles have to arrive simultaneously. In fact, according to NASA’s Glenn Research Center, “The actual velocity over the top of an airfoil is much faster than that predicted by the ‘Longer Path’ theory and particles moving over the top arrive at the trailing edge before particles moving under the airfoil” (emphases added).
Yes, the wing’s curved upper surface establishes an area of lower-pressure air above it, but there’s no venturi effect because there’s no venturi. Bernoulli doesn’t really tell us why this happens, only that it does. Bernoulli also doesn’t explain how non-cambered wing designs—those lacking a curved upper surface, or nearly so—can generate lift, or how symmetrical airfoils, with identical cambers on the top and bottom, also create it. And we haven’t even gotten to inverted flight.
As NASA says, “We can…use Bernoulli’s equation to compute the pressure, and perform the pressure-area calculation and the answer we get does not agree with the lift that we measure for a given airfoil. The lift predicted by the ‘Equal Transit’ theory is much less than the observed lift, because the velocity is too low. The actual velocity over the top of an airfoil is much faster than that predicted…and particles moving over the top arrive at the trailing edge before particles moving under the airfoil.”
Angle Of Attack
One way to explain inverted flight is Newton’s third law, that every action has an equal and opposite reaction. The simplest demonstration is to put your hand out the window of a moving automobile. By holding your hand horizontal relative to the oncoming air, there’s little resistance. Hold your hand perpendicular, however, and the moving air tends to push it rearward, toward the back of the car. You’ll need to flex your arm toward the front to keep it in place. If you hold your hand at, say, a 45-degree angle, it tends to want to move both rearward and upward at the same time. You need to flex your arm both forward and downward to counter the effect.
Your hand’s movement at the perpendicular and the 45-degree angle demonstrates Newton’s third law, regarding equal and opposite reactions: When the oncoming air meets your hand, it pushes up and/or aft. The same thing happens when a wing—or any other surface—is positioned at an angle not aligned with to the relative wind.
Thus, the inverted wing still generates lift by flying at an angle of attack greater than would be required when the cambered surface is the upper surface, thanks to Newton’s third law: as the air is pushed down by the wing, the Newtonian reaction also pushes up on the wing.
One thing to keep in mind when considering inverted flight is that a typical horizontal stabilizer is an airfoil. It’s mounted with the cambered surface facing down so its lift is directed opposite that of the wings as a counterbalance in favor of stability. So it’s not correct to say that lift only works in one direction. Presuming a cambered wing, the inverted airplane stays airborne more as a result of Newton’s third law than anything Bernoulli had to say.
Since lift is a force, Newton’s second law, which states that forces result from accelerating a mass, also figures prominently in our journey toward understanding it. The mass we’re concerned with is the air flowing past the airfoil as a fluid. As the air flows past the airfoil, some of it is deflected, or turned, which changes its velocity in magnitude, direction or both. At the leading edge, air is deflected upward and downward thanks to the airfoil’s shape. Also thanks to the airfoil, air is deflected downward as it passes beyond the trailing edge. Because of Newton’s third law involving equal and opposite reactions, the downward flow of air pushes the wing upward, creating lift.
One thing Newton’s laws regarding forces and reactions do not do, however, is explain why the relatively low-pressure air exists above the wing. Of course, neither does Bernoulli.
Co-Dependency
According to a February 2020 article by Scientific American (SA), aerodynamicists are aware of the gaps in theories of lift even as they apply ever-more advanced fluid dynamics computations. And they are moving toward what some might call a unified theory of lift.
One such aerodynamicist is Doug McLean, a former engineer at Boeing Commercial Airplanes and author of Understanding Aerodynamics: Arguing from the Real Physics. Part of McLean’s book is devoted to explaining lift and, as SA describes, he settled on four necessary components: “a downward turning of the airflow, an increase in the airflow’s speed, an area of low pressure and an area of high pressure.”
“They support each other in a reciprocal cause-and-effect relationship, and none would exist without the others,” his book is quoted by SA. “The pressure differences exert the lift force on the airfoil, while the downward turning of the flow and the changes in flow speed sustain the pressure differences.”
According to SA, McLean also came to realize his book “had not fully accounted for all the elements of aerodynamic lift, because he did not explain convincingly what causes the pressures on the wing to change from ambient.” McLean updated his text, recognizing that air is a fluid and that it interacts with a solid object. Fluid flows around objects are wildly variable in nature, requiring a separate discipline—fluid dynamics—and massive computing power to model the non-uniform behaviors.
Lift production has a broad impact on the air’s velocity and pressure as it comes in contact with the airfoil. There is a higher-pressure area below, a lower-pressure area above, and particles of air are accelerated and decelerated. Then, after the airfoil passes, velocity and pressure return to ambient. In other words, the science behind what actually happens when an airfoil generates lift is vastly more complicated than Bernoulli and Newton had tools to understand. Although the fluid dynamics field has made great advances, there are still minor details about lift production and the host of variables presented that elude the mathematicians.
For our purposes, few of those final details matter. What does matter is for us to understand that lift is produced by the complex interactions of a fluid (air) as it impacts solid objects (airfoils) or, if you prefer, vice versa. These interactions result in variable, dynamic combinations of pressure changes and downward-flowing air that produces lift.
The Sky Sucks
Several pearls of wisdom disguised as witty sayings have stuck with me from a test-preparation course I took when studying for the commercial and flight instructor written exams. One of them, referencing Bernoulli, is, “The sky sucks.” Another is, “If you have enough power, you can fly a brick.” The latter also highlights that this discussion of lift presumes a constant angle of attack and steady airspeed. In the real world, these values rarely are constant for very long and in any case depend heavily on how much power is both available and applied. The sidebar “What About Power?” below touches on power’s relationship to lift generation, which is itself another topic.
There are many subtopics of lift generation that are not addressed by this article, perhaps most notably how pressure differentials are distributed above and below the wing, and how their centers may move. When the centers of pressure move, so must the airplane’s attitude change, and vice versa. In part and for a given wing, these pressure differentials depend on angle of attack and airspeed, and remain important topics for understanding lift.
The punchline to all this is fairly simple: Generating lift is a complicated, dynamic process that depends on a handful of physical laws regarding pressure and force. While those laws are well-understood, their application can leave us with gaps in our understanding that are difficult to fill without embracing the interactions they define, which are themselves dependent on those same physical laws.

Newton’s Second And Third Laws
Sir Isaac Newton first published what has become known as his laws of motion in 1687. Newtonian physics works well to explain the behavior of objects moving at well below relativistic speeds, so using it on a piston single shouldn’t present a problem.
Newton’s Second Law
A popular translation of Newton’s second law is, “The alteration of motion is ever proportional to the motive force impressed; and is made in the direction of the right line in which that force is impressed.” This is the basic principle of acceleration: the speed of an object depends on the force applied.
Newton’s Third Law
This law often is described with the phrase “equal and opposite reaction” when an object is acted upon by an outside force. Its translation reads, “To every action there is always opposed an equal reaction: or the mutual actions of two bodies upon each other are always equal, and directed to contrary parts.”
Bad Bernoulli Takes
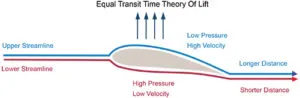
According to NASA, the “equal transit time” or “longer path” interpretation of Bernoulli’s principle states that “wings are designed with the upper surface longer than the lower surface, to generate higher velocities on the upper surface because the molecules of gas on the upper surface have to reach the trailing edge at the same time as the molecules on the lower surface.”
The error arises because, in reality, “the velocity on the upper surface of a lifting wing is much higher than the velocity which produces an equal transit time. If we know the correct velocity distribution, we can use Bernoulli’s equation to get the pressure, then use the pressure to determine the force. But the equal transit velocity is not the correct velocity.”
What About Power?

One of my least-favorite airplanes to witness—the AV-8B Harrier VTOL military aircraft, because it is loud—provides an extreme example of brick flying. Of course, a vertical Harrier takeoff involves no lift generation, only thrust exceeding the aircraft’s weight. Not until the Harrier’s wing is moving forward at what might be called a good clip is the downward vector from its single jet engine eliminated in favor of forward thrust.
To generate lift, we must have some form of energy, either kinetic (forward motion) or potential (altitude), or both in some combination. Regardless of which we use and in what proportion, the lift produced likely will change with the energy we apply to the task. In turn, control input also likely will be required to adjust the airplane’s attitude and compensate for increased lift from the wing and from the tail.
What The FAA Says

Unsurprisingly, materials published by the FAA don’t go into great detail on the relationships between Bernoulli’s principle and Newton’s laws. But they got the gist of it right. Consider the following excerpt from the FAA’s Pilot’s Handbook of Aeronautical Knowledge, FAA-H-8083-25B (PHAK):
“Applying Bernoulli’s Principle of Pressure, the increase in the speed of the air across the top of an airfoil produces a drop in pressure. This lowered pressure is a component of total lift. The pressure difference between the upper and lower surface of a wing alone does not account for the total lift force produced.
“The downward backward flow from the top surface of an airfoil creates a downwash. This downwash meets the flow from the bottom of the airfoil at the trailing edge. Applying Newton’s third law, the reaction of this downward backward flow results in an upward forward force on the airfoil.”
This article originally appeared in the April 2020 issue of Aviation Safety magazine.
For more great content like this, subscribe to Aviation Safety!




































Taught this stuff at university for years. Simple explanations almost always fail to describe things that depend on partial differential equations. Read on if you are math-curious. Ignore if math phobic or just don’t care.
For incompressible flow of a fluid with constant density {rho}, streamline curvature causes the pressure {p} in the fluid to decrease towards the center of curvature. Example: tornado vortex. The air pressure is minimum very near the center of curvature. We would also say that in the case of a vortex, there is a negative pressure gradient towards the center of curvature.
In engineering speak, we would say that streamline curvature creates a pressure gradient (+) in the direction opposite from the direction pointing towards center of curvature.
Define the direction pointing inward {n} towards the center of curvature of radius {r}, which is the surface of wing. Since the pressure far from the wing is atmospheric, and the pressure decreases towards the center of curvature, the pressure around the wing is less than atmospheric. Except of course at the stagnation point near the leading edge where one streamline is brought to a stop.
Writing Newton’s second law normal to a curved streamline, with the {n} direction being normal to the streamline pointing towards its fictitious center of curvature, we get:
{partial p} over {partial n} = – {rho v^2} over {r} (1)
A symmetrical airfoil produces no lift if the angle of attack {alpha} is zero. A positive alpha moves the stagnation point in the opposite direction of {alpha}, creating a smaller radius of curvature on the top of the wing than the bottom. In equation (1) smaller {r} means larger pressure gradient. Larger pressure gradient on the top of the wing than the bottom means lower pressure on the top than the bottom. Lift.
The reason this isn’t taught outside of fluid dynamics is because most people don’t like partial differential equations….
Here’s a reference with sketch and actual equations:
https://sbainvent.com/fluid-mechanics/forces-normal-to-a-streamline/
Jeb, as we’ve explained exclusively in Microlight Flying magazine, the truth is that the air molecules on the top of a wing all have heart attacks because they’re rushing to get to the back at the same time as the ones on the bottom, and lift is caused by the ones on the bottom going up to the funerals.
Hope that helps 🙂
I’ve flown radio-controlled model airplanes with flat-bottomed airfoils inverted many times over the years and they fly fairly well. Pretty much any relatively flat surface will produce lift as long as the airflow above it doesn’t separate and become turbulent. Something we probably don’t think about much is that our atmosphere at sea level is pushing against everything from all sides with about 14.7 PSI of force. Decrease it a little on one side while increasing it a little on the other can make a big difference. BTW, have you noticed how much the exhaust from a Falcon 9 rocket fans out as it gets higher? The higher it goes, the less atmosphere there is to keep its thrust from expanding.
I think a lot of your confusion might vanish if you watched this 1950’s (?) black-and-white video series by famed aerodynamicist Alexander Lippisch:
https://www.youtube.com/watch?v=V1oCDR3DBbo&list=PLo5KJaXac_mUq2WJcP0rsJP7aXy-lQxOA
It’s worth watching every minute of every video. I always wondered myself what it is that squeezes down on the molecules above the wing, forcing them to speed up; I won’t spoil it for you by telling you the answer.
Missing from the article – and from many of the standard texts on the subject – is that the air pressure on the bottom of the wing is (depending on angle of attack) also reduced from ambient. The Bernoulli diagrams and the one from the FAA in the article both show “high/increased” pressure below the wing in red, and “low/reduced” pressure above the wing. In reality, as the air accelerates across the wing, the pressure is reduced both above AND BELOW the wing. It’s just that it reduces more above the wing (longer journey, heart attacks, choose your own metaphor) than below, so there is a relative pressure difference providing a component of lift. But any pilot of a high wing fabric wing aircraft (especially one where the fabric is getting tired) will be able to confirm that the fabric on the bottom of the wing is often slightly concave between the ribs in flight, and not convex as the simplified diagrams would make you expect, even though the aircraft is flying completely normally. The aircraft is suspended by differential forces, there is no upward pressure on the wing. Alternatively I think that flight is just magic.
The fundamental problem with using Bernoullian and Newtonian physics is that they do not include all the terms needed to explain fluid flows occurring in flight. They are fine for simpler incompressible flows like water in pipes. For the past 50 years aerodynamicists have used the Navier-Stokes equations, which are five interrelated, partial-differential equations describing the conservation of mass, momentum and energy. Simplifications can be made for certain categories of flows, resulting in the Euler and potential equations, often combined with the boundary layer equations to account for friction in surfaces. (Swiss mathematicians Leonhard Euler and Daniel Bernoulli were close associates, mid 1700s). In the 70s-90s the N-S equations needed supercomputers to be solved for 3D flows. I know, as I was head of the CFD (Computational Fluid Dynamics) group at supercomputer maker Cray Research from 1984-1991. Today most airflows around wings and tail surfaces can be done on a modest PC, for instance using the program XFLR5. It uses the popular and well-validated airfoil program XFOIL from Mark Drela.
Thanks Jeb, great article! I would also like to see something generally like this to discuss the four forces of flight on a glider, where there is no thrust component. I get that the glider moves forward because it is descending. I can cold-drop a paper airplane and see it move forward. But I can’t find (in my mind) a vector chart for the glider where the lift is working against the drag vector. I can imagine the nose down and the lift is forward of vertical, but I can’t think of a way to make it anything but perpendicular to the relative wind. So what keeps the glider from slowing down? I know there’s an answer. I’m just failing to graph it out in my mind.
What makes a marble roll forward when a plane on which it rests is inclined? The marble has neither thrust nor lift. Nonetheless, tilt the plane, and suddenly the gravity on it has a forward component, which you could position as against acting against drag.
I’ve always been a Newton kinda guy instead of a Bernoulli fan. So simple and obvious. But doesn’t sound as intelligent and cool to explain. I kept quite around group discussion. I’ve always thought someone is overthinking that lift stuff.
It is all BS, we know what makes planes fly, it is money.
I thought it was FM.
I am sorry but the common pilot (and I hate to say many aero engineers) misconception about lift and Bernoulli is very much in evidence in this article. And to dismiss conservation of mass (the Kutta condition) out of hand for a non stalled airfoil is just folly.
As a simple mathematical proof you can derive Bernoulli from Newton’s laws. The problem is the when someone tries to use or say “v” is speed in the Bernoulli equation. This only works for a 1 dimensional problem since “v” is velocity and it is a vector. It has both speed and direction. For an airfoil section (2 dimensions) you still need speed AND direction.
So yes, Bernoulli works always and you do not need to make up things like having the air be even faster on top than the Kutta condition allows. But you must keep in mind and use the fact that velocity is a vector with both speed and direction. So even an infinitely thin airfoil can produce lift since while the speed is the same all over the airfoil the velocity is changing in direction. A change in direction is a change in velocity and this requires an acceleration. F=ma and lift is a force.
You can do a simple experiment to prove this to yourself. Put a flat sheet of paper on a table and blow over it horizontally. It will not lift. Curve the paper so the top is convex and do the same and it will lift. Or just hold them carefully in the air if you mistakenly think a “vacuum” is holding them to the table.
The air isn’t moving, the airfoil is… Smash the top of an airfoil into some air molecules that are just minding their own business and they are pushed upwards from the impact. In the space formally occupied by these air molecules is a vacuum. The bottom of the airfoil has a similar effect but, due to the angle of attack, lesser escape path and relatively flat surface, the air is compressed. I have no formulas to back this up but, they are not needed either. 🙂
Lift is very easy to explain.
When air molecules travel over the top of a wing surface that is inclined with respect to the airflow direction, they have to turn to fill the space behind the leading edge. The only force that is available to make them turn is the fact that all air molecules (above absolute zero) are constantly in random motion, and some, but not all, are traveling toward the space behind the wing. The portion of molecules that reach the wing surface exert a pressure on wing, but this pressure is lower than ambient atmospheric pressure so there is a net force pushing the wing up—or down, depending on the angle of incidence. Lift can work in the direction of the ground, obviously. Just not for very long. : )
Meanwhile on the “bottom” of the wing, molecules are bombarding the surface, which causes a higher pressure on the that surface. This force also pushes the wing “up.” It’s the combination of these two forces that create lift.
The air that passes over the wing and creates a down-flow of air at the trailing edge does not add to lift. That is an absurd misconception if you think about it. Those molecules have no connection to the wing. They are heading down because of their thermal motion and they happen to miss the wing, so they continue heading down. There is no mechanical linkage that would allow those molecules to do any work on the wing.
When a wing stalls, lift is approaching its maximum value, but it’s acting in an undesirable direction (i.e. it’s “lifting” the wing backwards).
Drag is lift in an undesired direction. Lift is drag in a desired direction.
Love this stuff, Jeb – thanks! Surprised not to see the “Coanda effect” here. Here’s a fun experiment:
Turn on the water in the sink, and hold the end of the handle of a spoon loosely between two fingers. Then, move the convex (bottom) of the spoon slowly into the stream of descending water, and what happens? The spoon will abruptly be “sucked” into the stream of water, propelled in that direction by the stream of water off the “trailing edge” of the spoon in the opposite direction – Newton!
My thinking on non-Bernoulli explanations on life was influenced by an article by a co-inventor of the Macintosh computer, the late Jef Raskin, an avid RC modeler, the title of which is, “The Coanda Effect; Understanding Why Wings Work”. I got the spoon experiment from him, though I’d observed such behavior since childhood. He also uses the Coanda effect to explain why ping pong balls, baseballs, etc. follow a curved path while moving through the air when rotating as well.
I like the fluid dynamics comments above. In the past couple of decades all the videos of aircraft flying through clouds, quite obviously pushing the atmosphere downwards in the process, reinforces one’s awareness of the Newtonian cause of lift.
What do you think?
Maybe all this airfoil stuff will stop all the GA accidents.
I have “flown” (launched really) many a cheap balsa glider with nothing but thin flat balsa wings and tail feathers that seem to glide just fine. Even the rubber-band powered ones fly well. These have zero camber and zero airfoil. I also used to fly control-line gas powered planes (with thicker wood), but these wings were also just flat wood.
I was always troubled by the explanation of air flowing faster over the upper wing surface due to the airfoil shape, because I believe there are some airfoils that have a symmetrical shape; so the air flow both over and under would be the same velocity.
Just the musings of a non-mathematician, non-physicist, non-scientist of any sort…
Alluded to, but simple. It’s all about flow turning. Any fluid turned away from a surface, typical of the upper side of the wing, reduces pressure. Any fluid turned into a surface, typical of the lower side of a wing, increases pressure. Local effects of a particular airfoil’s shape aside, the net force that usually wins the tug of war is directed upward for a wing in ‘normal’ flight. Predicting the magnitude of the lift is more work, but the airfoil is designed to optimize the turning of the flow which is beneficial to lift production at a given angle of attack. Forget the direction of the air in front or behind the wing. All it knows is the pressure exerted on the skin.
Now do water skis.
Something else that is often left out of the discussion. Bernoulli actually studied Newton’s work closely. Essentially Bernoulli’s work is an interpretation of Newton’s specifically tailored to hydrodynamics.
This discussion takes me back 50 years to my Senior year as a undergraduate Physics student. Undergrads were allowed to attend grad student seminars at lunch time. The best advice was to remain silent and appear stupid rather than open your mouth and remove all doubts! Well one time the Prof drew an airfoil on board and asked if anyone could explain why this shape generated lift. Having had lifelong love of aviation, having been a mechanic in the AF, and ground school perusing my pilot’s license, I knew I had this one cold. So I was asked up to explain. I told about air molecules having to move faster over top of wing causing lower pressure, which was a force that overcame gravity. The Professor asked how air molecules “knew” to move faster, was this some kind of “spooky action at a distance”. I knew I had got into a battle of witts and had just ran out of ammo. It wasn’t till later in grad school that I learned about Navier-Stokes equations and the circulation function that I realized just how little I really knew about fluid dynamics.
Are any of these THEORIES of any real use to the average pilot? We’ve all learned about the basics in school and aviation ground school, but in 60 years of flying, I haven’t found a need to express the theories–OR a practical use for needing to be able to display the theories on a chalkboard.
It’s kind of like RELIGION–either you believe, or you don’t–and the differences will be argued, fought over, and defended for millenia. (smile).
I’ve often found it helpful in discussing how airplanes fly to assert that “all the laws of physics have to be obeyed all the time”. For example, if the mass of the airplane is being accelerated upwards to counteract gravity, something else must be being accelerated downwards, and the only thing there is in the environment is air. So if you haven’t explained how air is accelerated downwards, you haven’t explained lift. (Old books used to show air leaving the wing horizontally, so they failed this test.) Likewise for pressure — if you haven’t explained why there is more force on the “bottom” of the wing than on the “top”, you haven’t explained how the airplane is being accelerated.